T.S. Eliot, who probably never thought about the chain rule while he was writing poetry. Photograph by Lady Ottoline Morrell. Public domain, via Wikimedia Commons.
“We shall not cease from exploration
And the end of all our exploring
Will be to arrive where we started
And know the place for the first time.”
—from Little Gidding [...]
Monthly archives for March, 2014
What T.S. Eliot Told Me about the Cha...
T.S. Eliot, who probably never thought about the chain rule while he was writing poetry. Photograph by Lady Ottoline Morrell. Public domain, via Wikimedia Commons.
“We shall not cease from exploration
And the end of all our exploring
Will be to arrive where we started
And know the place for the first time.”
—from Little Gidding [...]
Geometry and the Imagination
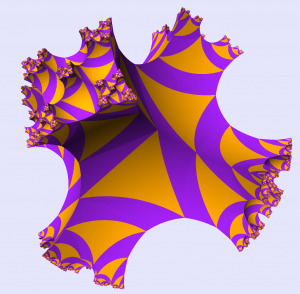The universal cover of a genus 3 handlebody, visualized using the program kleinian. Image: Danny Calegari.
If you like geometric group theory or amazing pictures (but especially geometric group theory), you might want to start reading Geometry and the Imagination, written by University of Chicago mathematician Danny Calegari. I’ve been follow [...]
A Different Pi for Pi Day
Nope, not this kind of pi(e). Image: flickr/djwtwo.
The symbol π is overloaded in math: depending on context and capitalization, π could be the constant we all know and love (or hate), a projection, a product, or a function. There’s plenty of stuff to read about the circle constant, so today I’m writing about one of those other π& [...]
On Teaching Analysis
Timothy Gowers, University of Cambridge mathematician and Fields Medalist, is teaching an analysis class this term, and fortunately for me, he’s blogging about it. Analysis IA is part of the first-year math major sequence at the University of Cambridge, and it is a rigorous approach to calculus at the undergraduate level. I am teaching a simi [...]
The Math Wars, Lewis Carroll Style
Lewis Carroll in 1863, photographed by Oscar Gustave Rejlander. Image: Public domain, via Wikimedia Commons.
Carroll’s book was a salvo in the “math wars” of the day, the subject of which was how best to teach geometry. Euclid was the standard textbook in private schools that taught mathematics, but many people found it wanting. To them, it w [...]
Chasing the Parallel Postulate
If you’d like to see these again, you’d better accept the parallel postulate. Image: Webber, via Wikimedia Commons.
Euclidean geometry, codified around 300 BCE by Euclid of Alexandria in one of the most influential textbooks in history, is based on 23 definitions, 5 postulates, and 5 axioms, or “common notions.” But as [...]
Recent Comments